Relative Motion

"There is no such thing on earth as an uninteresting subject; the only thing that can exist is an uninterested person"
G. K. Chesterton: Heretics (1905)

· Up to this point we have implicitly assumed that the co-ordinate system (reference frame) in which we describe our motion is at rest with respect to the earth. That is the observer is at rest on the earth.

· Suppose that the observer is moving at constant velocity with respect to the earth, for example the observer may be in a moving vehicle. What will be the velocity of the object as seen by this observer ? What is its relative motion ? (For example Monty Python's Galaxy Song)

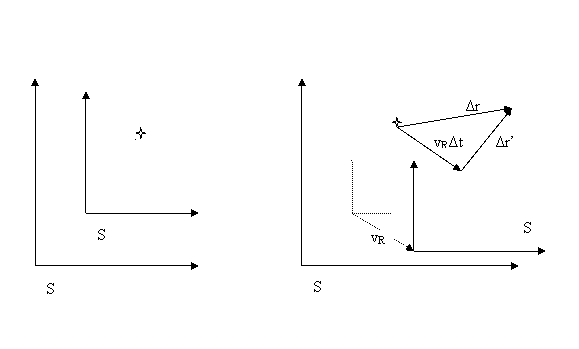
· In the example above we see that

The velocity in S is the vector sum of the velocity in S’ and the relative velocity of the two reference frames. This is the technical way of saying that velocities add. If I walk at 2 mph down the aisle of a plane traveling at 500 mph my velocity (relative to the earth) is either 502 mph or 498 mph.

· Assuming v_R is constant then differentiation of the above tells us that a = a’. The acceleration of an object has the same value when measured in two reference frames moving at constant velocity with respect to each other. This statement forms the basis of one of the postulates of the theory of relativity – the laws of Physics are the same when viewed in different reference frames moving at constant velocity relative to one another. Reference frames moving at constant velocity are often called inertial reference frames.

“Youth would be an ideal state if it came a little later in life”

Herbert Asquith – Observer (1923)

Dr. C. L. Davis
Physics Department
University of Louisville
email: c.l.davis@louisville.edu